Eddy Viscosity

This is a continuation of the Process Intensifier - Optimization with CFD: Part 1 paper.

The eddy viscosity can be understood to be an indication of a turbulence magnifier. Looking at relative magnitudes of this value give an indication of the level of turbulence contained within the enclosed volume. What becomes important is the size and magnitude of the volume. For this study, the 50% level of eddy viscosity was arbitrarily chosen as a discriminator to illustrate a concept. Hence, the iso-surface corresponding to that value was determined. It should be realized that contained within this volume is a higher turbulence measure, and outside the volume is lower. The implication to mixing becomes the size of the volume relative to how long particles are resident within.

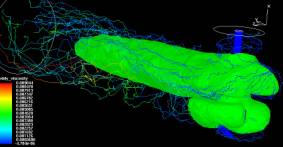
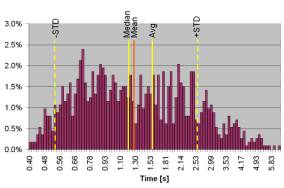
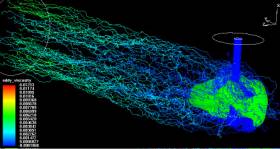
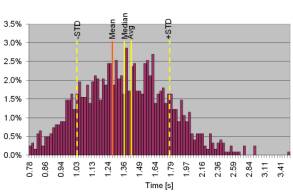
HGR – 650 GPM – 1750 RPM – Water	HGA – 650 GPM – 1750 RPM – Water
Figure 23: Characteristics of the HGR and HGA: Velocity vectors, mixing tracers, eddy viscosity, and RTD plots. Internals other than the impellers and shaft are not shown.

Figure 23 shows 4 different types of plots. The top and bottom ones have already been discussed. The second set is "mixing" tracer plots. Two sets of discrete tracer particles are injected to see how them mix. The blue comes from the beginning of the pipe and are uniformly distributed, just like the flow pattern tracers above. The green particles are added at the respective injection port. In the case of the HGR, it was below the lower impeller. In the case of the HGA, it was injected through a pipe just in front of each impeller. The colors this time have nothing to do with residence time. In both cases there is a fairly good mixture of these particles.

The 3rd set is the Eddy Viscosity plots. The light green object is the iso-surface of Eddy Viscosity. Everything inside the surface has a higher Eddy Viscosity and everything outside it will be less. The eddy viscosity has the same units as the kinematic viscosity, m2/s. This value when multiplied by the proper (Reynolds averages Navier Stokes - RANS) strain rate tensor produces the amount of "Reynolds shear" attributed to turbulence and then applied in the momentum equation (this is a very loose interpretation).

On the other hand, the size of the Eddy Viscosity iso-surface volume is much smaller for the two impellers of the LTA. Here is the case where two injection ports are upstream of the LTA. We can clearly see that some of the particles are completely missing these high-energy areas. Hence the poorer mixing. We can also see that the green particles (3 second residence times) are coming out of these surfaces. The maximum Eddy Viscosity of the LTA is only 0.006 m2/s. That means the green areas represent the surface where the values are 0.003 m2/s. This is much less than the other two examples. If we had chosen the same value, these surfaces would have been even smaller.

Even without an in-depth study of this characteristic, this shows that when mixing in a second component, it is best when it is injected within this high-energy volume. Obviously, larger volumes have a higher probability of capturing more particles, and larger volumes retain the particles longer. Perhaps the LTA would have worked better had the injection point been inside the green volume, just like the HGA.

Continue on with Non-Newtonian Xanthan Gum application  or  Go back to Results  or  Go back to the Title Page

Contact webmaster with questions or comments about this web site.
Copyright 2003 - 2013 Post Mixing Optimizations and Solutions, LLC
Last modified: February, 2013
Web Design by Sandy Gifford, Graphic Design, Web Development, and Illustration